The Chemist Volume 90 | Number 1
ISSN 1945-0702
Phase Equilibria in the Tl2Te-Tl5Te3-Tl9SmTe6 System Samira Zakir Imamaliyeva*, Vagif Akber Gasymov, Mahammad Baba Babanly Institute of Catalysis and Inorganic Chemistry named after acad. M. Nagiyev National Academy of Science of Azerbaijan, H. Javid Ave., 131, Az-1143, Baku, Azerbaijan (*E-mail: samira9597a@gmail.com)
Abstract: Phase equilibria in the Tl2Te-Tl5Te3-Tl9SmTe6 system were experimentally studied by means of differential thermal analysis, powder X-ray diffraction technique and microhardness measurements applied to equilibrated alloys. Several isopleth sections and isothermal section at 300 K, as well as projections of the liquidus and solidus surfaces, were constructed based on the experimental data. It was established that homogeneity area of solid solutions with Tl5Te3 structure (δ-phase) occupied more than 90% of the concentration triangle. A narrow area of solid solutions (α-phase) based on Tl2Te was detected. Key Words: Thallium-samarium tellurides, phase equilibria, solid solutions, crystal structure.

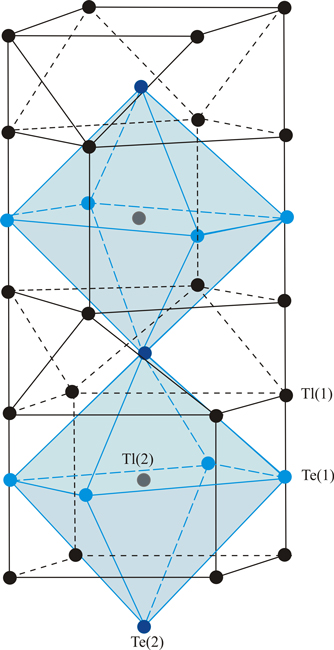
Introduction

Chalcogenides of heavy p-elements have received a lot of attention thanks to their interesting functional properties, such as thermoelectric, photoelectric, optical, magnetic  properties [1-3]. Furthermore, in recent years some of such compounds have attracted both scientific and technological interest as topological insulators [4-6]. Doping by rare-earth elements can improve their properties and give them additional functionality, such as the magnetic properties [7,8].

Thallium subtelluride, Tl₅Te₃ is suitable "matrix" for creation of new complex materials. This compound crystallizes in tetragonal structure [9] (Sp.gr.I4/mcm) with four formula units per unit cell (Fig.1). The basic structural component of Tl₅Te₃ compound is octahedron with a thallium atom, Tl(2), in its center. These octahedra connected by vertices form a frame Tl₄Te₁₂, or (TlTe₃)₄. The other 16 thallium atoms, Tl(1) link octahedra along the c axis and form a unit cell Tl₁₆(TlTe₃)₄. B³⁺ (B³+-Sb, Bi) substitution for half of the Tl(2) atoms, resulting  in the compounds Tl₉BTe₆ or Tl₁₆[(T1¹⁺_0,5B³⁺_0,5)Te₃]₄(I), while  the replacement of all these thallium atoms by cations A²⁺ (A²⁺-Sn, Pb) leading to formation of Tl₄ATe₃ or Tl₁₆[A²+Te₃]₄ (II).  

Fig 1. Basic structural component of Tl5Te3 compound.

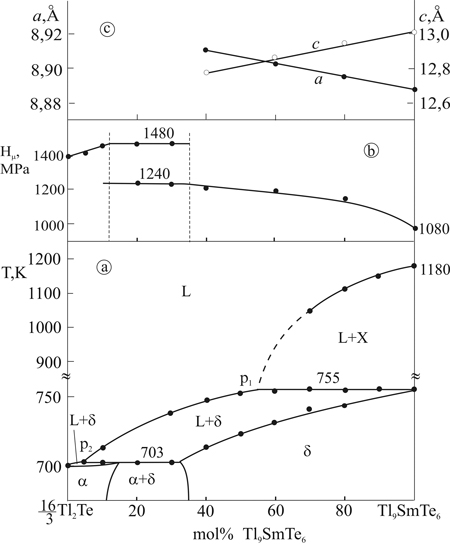
Compounds of type (I) and (II) were detected during the experimental phase equilibria studies of respective ternary systems [10-13]. These materials possess thermoelectric properties, and Tl₉BiTe₆ was found to have the highest ZT value [1,2,14].

A new thallium lanthanide tellurides with composition Tl₉LnTe₆ (Ln-Ce, Nd, Sm, Gd, Tm, Tb) were obtained first by Babanly M.B. [15-17]. It was shown that above mentioned compounds are substitution variants of Tl₅Te₃, and their melting character and crystal lattices parameters were determined. Moreover, ytterbium does not form the compound Tl₉YbTe₆ [17, 18]. Later, the crystal structure as well as magnetic and thermoelectric properties for a number Tl₉LnT₆-type compounds were determined by authors [19-21]. 

According to the phase diagrams [10-13, 15], all listed ternary compounds are phases with variable composition and have wide homogeneity areas.

In our previous papers [22-25], we have presented the results of phase equilibria investigations of the Tl₅Te₃-Tl₉NdTe₆-Tl₉BiTe₆, Tl₅Te₃-Tl₄PbTe₃-Tl₉NdTe₆, Tl₉NdTe₆-Tl₉BiTe₆-Tl₄PbTe₃, Tl₂Te-Tl₉NdTe₆-Tl₉BiTe₆, and Tl₂Te-Tl₉TbTe₆ systems including Tl₅Te₃ compound or its structural analogues. We found that the former three systems are characterized by formation of continuous solid solutions and the latter two systems- by wide areas of solid solutions.

Here we represent a detailed investigation of phase relationships of the Tl-Sm-Te system in the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ composition area.

Tl₂Te and Tl₅Te₃ compounds melt congruently at 698 and 723 K and form the eutectic (695 K, ~ 34 at.% Tl) [26]. These data were confirmed by Okamoto [27]. Tl₂Te crystallizes in the monoclinic system (space group C2/c; a = 15.662; b = 8.987; с=31.196 Å, β=100.76⁰, z=44) [28], while tetragonal lattice parameters of Tl₅Te₃  are equal to a = 8.930; c = 12.598 Å [9]. Tl₉SmTe₆ melts with decomposition by the peritectic reaction at 755 K and has lattice constant: a=8.888; c=13.013 Å, z=2 [16].

Experimental

Materials and Syntheses

Starting compounds Tl₂Te and Tl₅Te₃ were synthesized by melting of high purity elements in evacuated (~ 10^-2 Pa) quartz ampoules at 750 K with following slow cooling. Tl₉SmTe₆ was synthesized at 1000 К using the ceramic method. Taking into account the incongruent melting of Tl₉SmTe₆ [16], this compound was annealed at 700 K for 300 h after the synthesis.

The purity of the synthesized compounds was checked by differential thermal analysis (DTA) and powder X-ray diffraction (XRD) techniques.

Alloys of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system were prepared by melting the stoichiometric quantities of the pre-synthesized binary and ternary compounds in evacuated silica ampoules at 900 K in a tube furnace. After the synthesis, alloys were powdered in an agate mortar, pressed into pellets and reheated at 680 K within 1000 h. In order to prevent a reaction between the ampoules and samarium, the silica tubes were coated with a carbon film via the decomposition of ethanol.

Methods

DTA and XRD analyses as well as microhardness measurements were employed to analyze the samples.
DTA was performed using a NETZSCH 404 F1 Pegasus differential scanning calorimeter. The crystal structure was analyzed by a powder X-ray diffraction technique at room temperature using a Bruker D8 diffractometer utilizing CuKa radiation within 2θ = 10 to 70⁰. Microhardness measurements were done with a microhardness tester PMT-3, the typical loading being 20g.

Results & Discussion

The combined analysis of experimental data enabled us to construct the self-consistent diagram of the phase equilibria in the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system (Table, Fig.2-7).

The (16/3)Tl₂Te-Tl₉SmTe₆ system (Fig.2) is a part of the Tl₂Te-Sm₂Te₃ system. It is a non-quasi-binary because of the incongruent character of the Tl₉SmTe₆ melting. However, it behaves as a quasi-binary system below the peritectic horizontal at 755 K. The phase diagram is characterized by formation of a wide area of solid solutions (δ) with the Tl₅Te₃ structure. Liquidus consists of three curves corresponding to the primary crystallization of α;- and δ- phases based on Tl₂Te and Tl₉SmTe₆, as well as the unknown infusible X phase (presumably TlSmTe₂). Horizontals at 755 and 703 K correspond to peritectic equilibria L+X<->δ and L+δ<->α. The peritectic points p₁ and p₂ correspond to 65 and 5 mol% Tl₉SmTe₆, respectively.

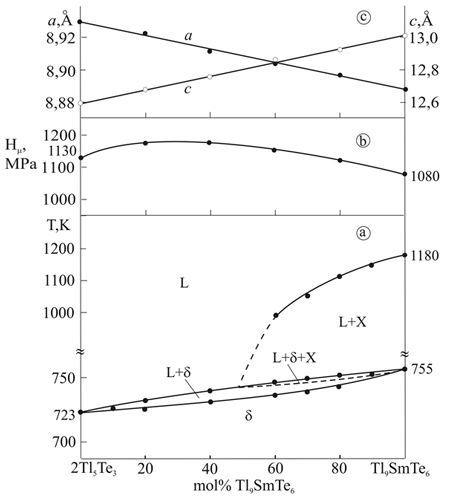
The equilibrium phase diagram of the 2Tl₅Te₃-Tl₉SmTe₆ system (Fig.3) is also non-quasi-binary due to peritectic melting of Tl₉SmTe₆ compound. This system is characterized by the formation of a continuous series of solid solutions (d) based on Tl₅Te₃. The δ-solid solutions primarily crystallize in 0-65 mol% Tl₉SmTe₆ composition area; whereas in region more than 65 mol% Tl₉SmTe₆, the X phase crystallizes. In this composition area below 755 K, a three-phase area L+X+δ should be formed as the result of monovariant peritectic reaction L+X<->δ. However, this area is not experimentally fixed due to narrow temperature interval and shown by dashed line (Fig.3a).

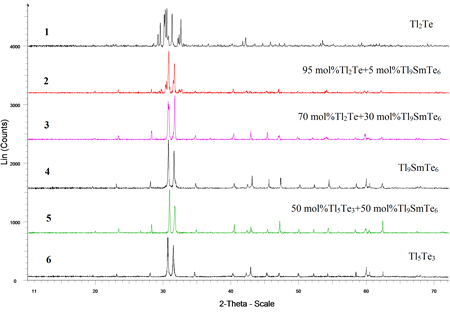
The results of the microhardness measurements are in agreement with constructed phase diagram (Figs.2b and 3b). For the Tl₅Te₃-Tl₉SmTe₆ system, a curve has a flat maximum (Fig.3b), which is typical for systems with continuous solid solutions. For the Tl₂Te-Tl₉SmTe₆ system, the microhardness values of starting compounds are increased within homogeneity areas of α- and δ-phases, and remain constant in the α+δ two-phase region (Fig.2b).

Powder X-ray analysis data confirm the phase diagrams of the above-mentioned systems (Fig.4). For the Tl₅Te₃-Tl₉SmTe₆ system, powder diffraction patterns of starting compounds and intermediate alloys are qualitatively similar with slight reflections displacement from one compound to another (Fig.4, diffraction patterns 4-6). For example, we present the powder diffraction pattern of alloy with 50 mol% Tl9SmTe6. Solid solutions obey the Vegard’s law, i.e. the lattice parameters depend linearly on composition. In the Tl₂Te-Tl₉SmTe₆ system, the alloys with compositions ³30 mol% Tl₉SmTe₆ are monophasic with Tl₅Te₃-type diffraction patterns (Fig.4, diffraction pattern 3), while alloy with 25mol% Tl₉SmTe₆ composition is bi-phasic. Besides the δ-phase reflections this alloy contains weak peaks of δ-phase (Fig.4, diffraction pattern 2).

Fig 4. XRD patterns for different compositions in the Tl2Te-Tl9SmTe6 (patterns 1-4) and Tl5Te3-Tl9SmTe6 (patterns 4-6) systems.

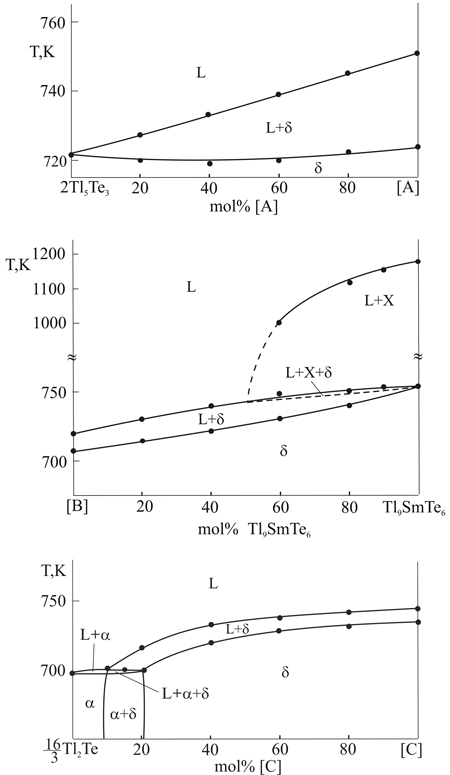
Isopleth sections of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system (Fig.5).

Figs. 5a-c show the isopleth sections Tl₅Te₃-[A], Tl₉SmTe₆-[B] and Tl₂Te-[C] of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system, where A, B and C are alloys from the respective boundary system. As can be seen, over the entire compositions range of the Tl₅Te₃-[A] system only δ-phase crystallizes from the melt.  

Fig 5. Polythermal sections 2Tl5Te3-[A], Tl9SmTe6-[B] and (16/3)Tl2Te-[C] of the phase diagram of the Tl2Te-Tl5Te3-Tl9SmTe6 system.

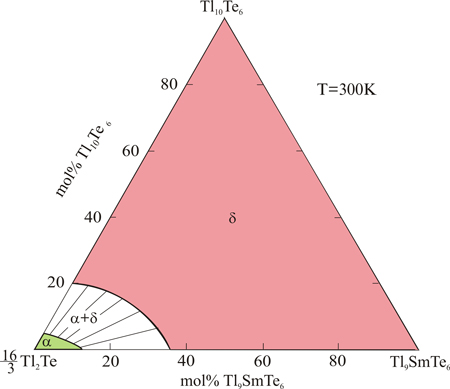
According to the phase diagram of the Tl₉SmTe₆-[В] section in the composition area <50 mol% Tl₉SmTe₆, the primary crystallization of the δ-phase occurs from the liquid phase. In the Tl₉SmTe₆- rich alloys the X-phase first crystallizes, then a monovariant peritectic equilibrium L+X<->δ takes place.

The liquidus of Tl₂Te-[C] section consists of two curves of primary crystallization of α- and δ-phases. The intersection point of these curves corresponds to the monovariant peritectic reaction L+δ<->α (703 K). Below the solidus, this section passes through the α, α+δ and δ phase areas.

The isothermal sections of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system at 300 K (Fig.6)

The isothermal sections of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system at 300 K (Fig.6) consists of three phase areas. Over 90% of the concentration triangle is occupied by δ-solid solutions with Tl₅Te₃ structure. α-phase based on Tl₂Te has a narrow homogeneity area in the corresponding angle of the triangle. Homogeneity areas of the α- and δ-phases are separated by α+δ two-phase region.

Fig 6. Isothermal section of the phase diagram of the Tl2Te-Tl5Te3-Tl9SmTe6 system at 300 K.

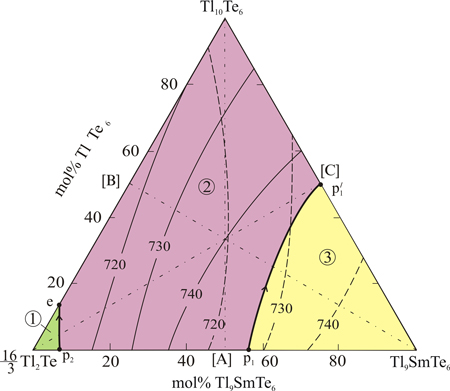
The liquidus surface projection (Fig.7)

Liquidus of Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system consists of three  fields  of  the  primary  crystallization  of  α-,δ-  and Х-phases.These fields are separated by p₂e and p₁p₁' lines, which correspond to the monovariant peritectic equilibria L+δ<->α and L+Х<->δ. Near the eutectic point (e) the peritectic equilibrium L+δ<->α must be transformed into L<->α+δ eutectic equilibrium. However, coordinates of this transformation are not experimentally fixed due to narrow temperature range. Solidus surface consists of two areas corresponding to the completion of crystallization α- and δ-phases.  

Fig 7. Projection of the liquidus and solidus (dashed lines) surfaces of the Tl2Te-Tl5Te3-Tl9SmTe6 system. Primary crystallization fields of phases: 1-α; 2-δ; 3-X. Dash-dot lines show the investigated sections.

Conclusion

A complete T-x-y diagram of the Tl₂Te-Tl₅Te₃-Tl₉SmTe₆ system is constructed, including the T-x diagrams of boundary systems Tl₅Te₃-Tl₉SmTe₆ and Tl₂Te-Tl₉SmTe₆, some isopleth sections, isothermal section at 300 K and liquidus and solidus surface projections. Studied system is characterized by the formation of wide field of δ-solid solutions with the Tl₅Te₃ structure, occupying more than 90% of the concentration triangle. Obtained experimental data can be used for choosing the composition of solution-melt and for determining of temperature conditions for growing crystals of δ- phase with a given composition.

Acknowledgment

The work was supported by the Science Foundation of the State Oil Company of Azerbaijan Republic (Grant for the project "Preparation and investigation of new functional materials based on complex metal chalcogenides for alternative energy sources and electronic engineering", 2014).

References

1. Shevelkov AV. Russ. Chem. Rev, 2008, 77, 1.
2. CRC Handbook of Thermoelectrics, ed. by D. M. Rowe. CRC Press, New York, 1995, 701p.
3. Koc H, Simsek S, Mamedov AM & Ozbay E. Ferroelectrics, 2015, 483(1), 43.
4. Niesner D, Otto S, Hermann V, Fauster Th, Menshchikova TV, Eremeev SV, Aliev ZS, Amiraslanov IR, Echenique PM, Babanly MB, Chulkov EV. Phys.Rev.B, 2014, 89, 081404.
5. Politano A, Caputo M, Nappini S, Bondino F, Aliev ZS, Babanly MB, Chulkov EV. J.Phys.Chem.C, 2014, 118, 21517.
6. Yan B, Zhang H-J, Liu C-X, Qi X-L, Frauenheim T and Zhang S-C. Phys. Rev.B, 2010, 82, 161108(R).
7. Alemi A, Klein A, Meyer G, Dolatyari M and Babalou AZ. Anorg. Chem, 2011, 637, 87.
8. Wu F, Song H, Jia J, Xu H. Prog.Nat.Sci, 2013, 23 (4), 408.
9. Schewe I, Böttcher P, Schnering HG. Z.Kristallogr, 1989, Bd188, 287.
10. Babanly MB, Akhmad'ar A, Kuliev AA. Russ. J. Inorg. Chem, 1985, 30, 1051.
11. Babanly MB, Akhmad'yar A, Kuliev AA. Russ.J.Inorg. Chem, 1985, 30, 2356.
12. Babanly MB, Gotuk A A, Kuliev AA. Inorg.Mater, 1979, 15, 1011.
13. Gotuk AA, Babanly M B, Kuliev AA. Inorg. Mater, 1979, 15, 1062.
14. Wolfing B, Kloc C, Teubner J, Bucher E. Phys. Rev. Let, 2001, 36 (19), 4350.
15. Imamaliyeva SZ, Sadygov FM, Babanly MB. Inorg.Mater, 2008, 44, 935.
16. Babanly MB, Imamaliyeva SZ, Babanly DM. Azerb.Chem.J, 2009, 2, 121.
17. Babanly MB, Imamaliyeva SZ, Sadygov FM. News of BSU. Nat. Sci.Ser, 2009,  4, 5.
18. Imamaliyeva SZ, Mashadiyeva LF, Zlomanov VP, Babanly MB. Inorg.Mater, 2015, 51, 1237.
19. Bangarigadu-Sanasy S, Sankar C R, Assoud A,  Kleinke H. Dalton Trans, 2011, 40, 86.
20. Bangarigadu-Sanasy S, Sankar C R, Schlender P, Kleinke H. J. Alloys Compd, 2013, 549, 126.
21. Bangarigadu-Sanasy S, Sankar CR, Dube PA, Greedan JE, Kleinke H. J.Alloys. Compd, 589, (2014) 389. 
22. Babanly MB, Tedenac J-C., Imamaliyeva SZ, Guseynov FN, Dashdieva GB.  J.Alloys Compd, 2010, 491, 230.
23. Imamaliyeva SZ, Guseynov FN, Babanly MB. J. Chem. Probl, 2008, 4, 640. 
24. Imamaliyeva SZ, Guseynov FN, Babanly M. Azerb.Chem.J, 2009, 1, 49.
25. Imamaliyeva SZ, Gasanly TM, Sadygov FM, Babanly MB. Azerb.Chem.J, 2015, 3, 93.
26. Asadov MM, Babanly MB, Kuliev AA. Inorg. Mater, 1977, 13(8), 1407.
27. Okamoto H. J. Phase Equilib, 2001, 21(5), 501.
28. Cerny R, Joubert J, Filinchuk Y, Feutelais Y. Acta Crystallogr. C, 2002, 58(5), 163.